5 Ways Youngs Inequality

The concept of Young's inequality is a fundamental principle in mathematics, particularly in the field of functional analysis and inequality theory. It has far-reaching implications in various areas, including calculus, linear algebra, and optimization techniques. Young's inequality is named after the mathematician William Henry Young, who first introduced it in the early 20th century. In this article, we will delve into the concept of Young's inequality and explore its significance in mathematics.

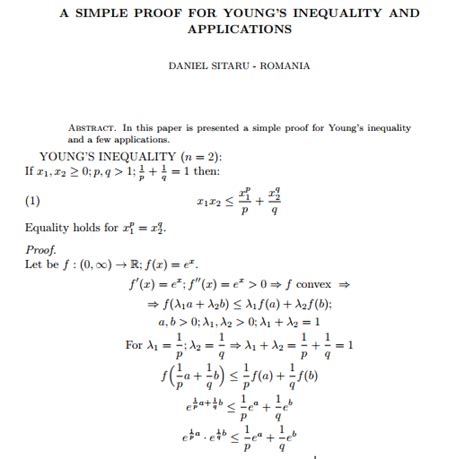
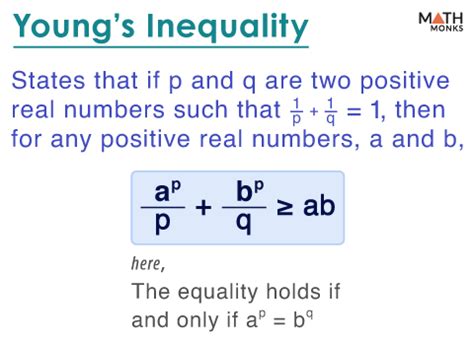
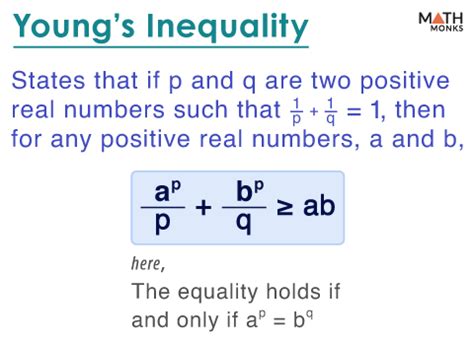
Young's inequality states that for any non-negative real numbers $a$ and $b$, and any positive real numbers $p$ and $q$ such that $\frac{1}{p} + \frac{1}{q} = 1$, the following inequality holds: $ab \leq \frac{a^p}{p} + \frac{b^q}{q}$. This inequality has numerous applications in mathematics, physics, and engineering, and is a crucial tool for establishing bounds and limits in various mathematical contexts.

Introduction to Young's Inequality

The concept of Young's inequality is closely related to the idea of convexity and concavity of functions. In essence, it provides a way to bound the product of two non-negative numbers in terms of their $p$-th and $q$-th powers, respectively. This has significant implications in optimization problems, where one needs to maximize or minimize a function subject to certain constraints.

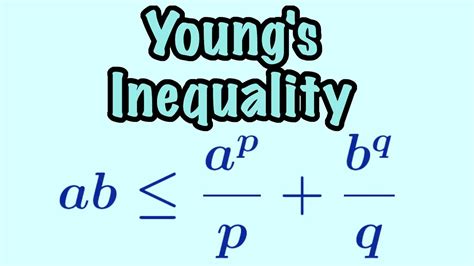
Applications of Young's Inequality

Young's inequality has numerous applications in mathematics, physics, and engineering. Some of the key areas where Young's inequality is used include: * Calculus: Young's inequality is used to establish bounds and limits in calculus, particularly in the context of optimization problems. * Linear algebra: Young's inequality is used to bound the norm of a matrix product in terms of the norms of the individual matrices. * Optimization techniques: Young's inequality is used to establish bounds and limits in optimization problems, particularly in the context of convex optimization.

Proof of Young's Inequality

The proof of Young's inequality is based on the concept of convexity and concavity of functions. Specifically, it uses the fact that the function $f(x) = x^p$ is convex for $p \geq 1$, and the function $g(x) = x^q$ is concave for $q \leq 1$. By using the definition of convexity and concavity, one can establish the inequality $ab \leq \frac{a^p}{p} + \frac{b^q}{q}$.

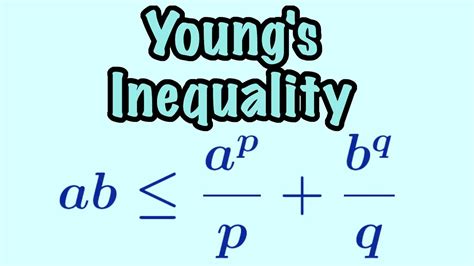
Generalizations of Young's Inequality

Young's inequality has been generalized in various ways, including: * Weighted Young's inequality: This inequality involves weighted sums of $a$ and $b$, and is used in applications such as data analysis and signal processing. * Multivariate Young's inequality: This inequality involves multiple variables, and is used in applications such as optimization and control theory. * Vector-valued Young's inequality: This inequality involves vector-valued functions, and is used in applications such as functional analysis and partial differential equations.

Conclusion and Future Directions

In conclusion, Young's inequality is a fundamental principle in mathematics, with far-reaching implications in various areas, including calculus, linear algebra, and optimization techniques. Its generalizations, such as weighted Young's inequality, multivariate Young's inequality, and vector-valued Young's inequality, have significant applications in data analysis, signal processing, optimization, and control theory. Future research directions include exploring new applications of Young's inequality, developing new generalizations and extensions, and investigating the relationships between Young's inequality and other mathematical concepts.

Young's Inequality Image Gallery

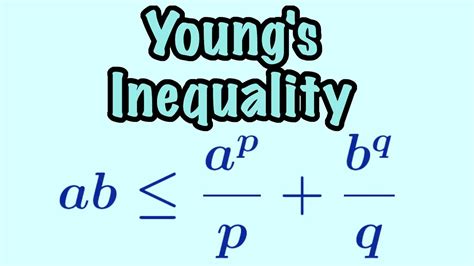
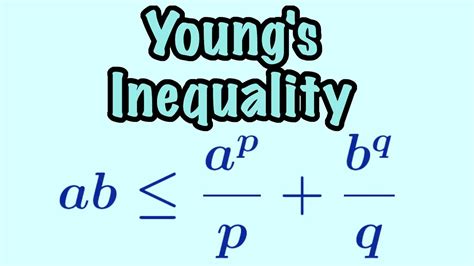
What is Young's inequality?

+

Young's inequality is a mathematical principle that states that for any non-negative real numbers $a$ and $b$, and any positive real numbers $p$ and $q$ such that $\frac{1}{p} + \frac{1}{q} = 1$, the following inequality holds: $ab \leq \frac{a^p}{p} + \frac{b^q}{q}$.

What are the applications of Young's inequality?

+

Young's inequality has numerous applications in mathematics, physics, and engineering, including calculus, linear algebra, optimization techniques, data analysis, and signal processing.

What are the generalizations of Young's inequality?

+

Young's inequality has been generalized in various ways, including weighted Young's inequality, multivariate Young's inequality, and vector-valued Young's inequality, which have significant applications in data analysis, signal processing, optimization, and control theory.

How is Young's inequality used in optimization problems?

+

Young's inequality is used to establish bounds and limits in optimization problems, particularly in the context of convex optimization, where one needs to maximize or minimize a function subject to certain constraints.

What are the future directions for research on Young's inequality?

+

Future research directions include exploring new applications of Young's inequality, developing new generalizations and extensions, and investigating the relationships between Young's inequality and other mathematical concepts.

We hope this article has provided a comprehensive overview of Young's inequality and its significance in mathematics. If you have any questions or would like to learn more about this topic, please don't hesitate to comment or share this article with others.